Answer:
Step-by-step explanation:
The first question asks for the object's height. That is a y-dimension thing so we will use the one-dimensional formula:
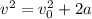 Δx and we are looking for delta x, which is the displacement of the object (here, the height since displacement in the y-dimension is height). Before we do that, though, we need to know the initial upwards velocity, which is found in:
Δx and we are looking for delta x, which is the displacement of the object (here, the height since displacement in the y-dimension is height). Before we do that, though, we need to know the initial upwards velocity, which is found in:
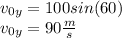
And we also have to know from previous physics experience that the final velocity of an object at its max height is 0. Filling in:
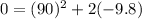 Δx
Δx
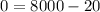 Δx and
Δx and
-8000 = -20Δx so
Δx = 400 m
Now for the time it was in the air...we will use the simple equation

So the object is in the air for 9 seconds. Now we go to the other dimension, the x-dimension, to find out how far it goes horizontally. We will use the equation:
Δx =
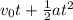 but first we have to find the horizontal velocity, which is NOT the same as the upwards velocity.
but first we have to find the horizontal velocity, which is NOT the same as the upwards velocity.

And another thing we need to know prior to solving problems like this is that the acceleration in the x-dimension is ALWAYS 0. Filling in the equation:
Δx =
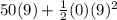
Δx = 500 m