Answer:
a went to 5a in the larger square
Explanation:
The area of a square with a side m is given by
 , since it's sides are all equal. Thus, a square with area
, since it's sides are all equal. Thus, a square with area
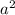 has sides of a length. The larger square has area 25
has sides of a length. The larger square has area 25
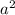 . A side is
. A side is
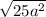 , or 5a.
, or 5a.
The side of the smaller square, a, was lengthened by a factor of 5, to 5a.