Question

Find the average rate of change for the function f(x) = 2 ^ x + 49 Using the intervals of x = 4 to x = 7 Show ALL your work to receive credit

Answer 1

48

Explanation:

We are given the exponential function:—

`\displaystyle \large{f(x)=2^x+49}`

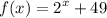
Finding average rate of change from x = 4 to x = 7:—

The formula to find average rate of change:—

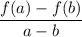`\displaystyle \large{(f(a)-f(b))/(a-b)}` or
`\displaystyle \large{(f(x+h)-f(x))/(h)}`

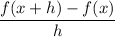
Let a be 7 and b be 4, therefore:—

`\displaystyle \large{(f(7)-f(4))/(7-4) = ((2^7+49)-(2^4+49))/(3)}`

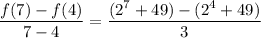
Evaluate:—

`\displaystyle \large{((128+49)-(16+49))/(3)}\\\displaystyle \large{(128+49-16-49)/(3)}\\\displaystyle \large{(128+16)/(3)}\\\displaystyle \large{(144)/(3)}\\\displaystyle \large{48}`

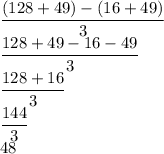
Therefore, the average of change from x = 4 to x = 7 is 48.