Question

A store is having a sale on jelly beans and trail mix. For 7 pounds of jelly beans and 9 pounds of trail mix is a total of $42. For 5 pounds of jelly beans and 3 pounds of trail mix is a total of $24. Find the cost for each pound of jelly beans and each pound of trail mix?

Answer 1

One pound of jelly beans costs $3.75.

One pound of trail mix costs $1.75.

Explanation:

Let
`x` be equal to one pound of jelly beans.

Let
`y` be equal to one pound of trail mix.

1) Form equations

We're given:

7 pounds of jelly beans + 9 pounds of trail mix = $42

⇒
`7x+9y=42`

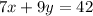
5 pounds of jelly beans + 3 pounds of trail mix = $24

⇒
`5x+3y=24`

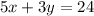
Now, we have our two equations:

`\displaystyle\left \{ {{7x+9y=42} \atop {5x+3y=24}} \right.`

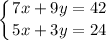
2) Solve for x using elimination

`\displaystyle\left \{ {{7x+9y=42} \atop {5x+3y=24}} \right.`

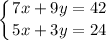
Multiply the second equation by 3 to change 3y to 9y:

`5x+3y=24\\3(5x+3y)=3(24)\\15x+9y=72`

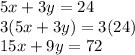
Subtract the first equation from the second to eliminate y:

`15x+9y=72\\- 7x+9y=42\\\rule{2.5cm}{0.4pt}\\8x=30`

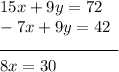
Divide both sides by 8 to isolate x:

`\displaystyle x=(30)/(8) \\\\x=3.75`

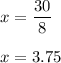
Therefore, one pound of jelly beans costs $3.75.

2) Solve for y using substitution

`\displaystyle\left \{ {{7x+9y=42} \atop {5x+3y=24}} \right.`

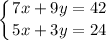
Now, we know that x=3.75. Plug this value into one of the equations and solve for y:

`5x+3y=24\\5(3.75)+3y=24\\18.75+3y=24\\3y=24-18.75\\3y=5.25\\\\\displaystyley=(5.25)/(3) \\\\y=1.75`

Therefore, one pound of trail mix costs $1.75.

I hope this helps!