Answer:
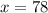
Explanation:
Skills needed: Exterior Angle Theorem, Triangle Geometry:
1) We need to solve for
 and there are 2 methods to do it. I will go over the fastest way (since it's better).
and there are 2 methods to do it. I will go over the fastest way (since it's better).
- This involves the exterior angle theorem (see the image attached to see how that works.
2) In order to use this theorem, we need to solve for the angle near
 . This angle would be
. This angle would be
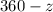 , which is
, which is
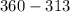 (since
(since
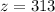 ).
).
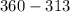 =
=
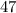
3) Then, we can use the fact that
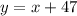 (47 is the measure of the angle we just solved for.)
(47 is the measure of the angle we just solved for.)
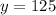 , so
, so
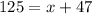
Subtract 47 from both sides, and get
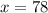
Hope you understood and have a nice day! :D
Note: I have attached one image that shows my work, and another image that shows the exterior angle theorem (which I created on a whiteboard). Hope you get it! If you have any questions, comment and I can answer it ASAP!