Answer:
CD = 38
Explanation:
Since AB = 3x + 2, CD = 3x + 2
To solve for the length of CD, we'll need to solve for x first by using the perimeter formula
Solving for x...
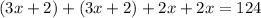
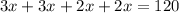
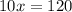
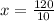
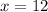
Now that we know what x equals, plug in x into the expression of side CD
CD = 3x + 2
CD = 3(12) + 2
CD = 36 + 2
CD = 38
Therefore, the length of side CD = 38
-------------------------------------------------------
Check:
AB = CD | BC = DA
38 + 38 for AB and CD
BC + DA = 2x + 2x
BC + DA = 2(12) + 2(12)
BC + DA = 24 + 24
BC + DA = 48
38 + 38 + 48 = perimeter
perimeter = 124