Answer:
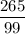
Explanation:
Hi there!
Let
 be equal to the fraction form of the decimal.
be equal to the fraction form of the decimal.

Multiply both sides by 100:
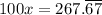
The reason we multiply both sides by 100 is that 2.6767... has two repeating decimals, 6 and 7, and 100 has two zeros.
Now, we have these two equations:
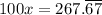

Subtract
 from
from
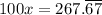 :
:
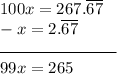
Isolate
 by dividing both sides by 99:
by dividing both sides by 99:
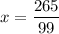
I hope this helps!