Question

A sample of oxygen occupied 568 ml. when the pressure increased to 911.6 mm Hg. at constant temperature, what volume did the gas initially occupy when the pressure was 248.71 mm Hg?

a 2082
b 155.0
c 2114
d 399.2

Answer 1

`\boxed {\boxed {\sf A. \ 2082 \ mL}}`

Step-by-step explanation:

We are asked to find the initial volume of a gas given a change in pressure. Since the temperature remains constant, we are only concerned with volume and pressure. We will use Boyle's Law, which states the volume of a gas is inversely proportional to the pressure. The formula for this law is:

`P_1V_1= P_2V_2`

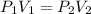
The pressure was initially 248.71 millimeters of mercury, but the volume is unknown.

`248.71 \ mm \ Hg * V_1 = P_2V_2`

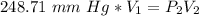
The pressure is increased to 911.6 millimeters of mercury and the volume is 568 milliliters.

`248.71 \ mm \ Hg * V_1 = 911.6 \ mm \ Hg * 568 \ mL`

We are solving for the initial volume, so we must isolate the variable V₁. It is being multiplied by 248.71 millimeters of mercury. The inverse operation of multiplication is division, so we divide both sides by 248.71 mm Hg.

`\frac {248.71 \ mm \ Hg * V_1 } {248.71 \ mm \ Hg}= (911.6 \ mm \ Hg * 568 \ mL )/(248.71 \ mm \ Hg)`

`V_1 = (911.6 \ mm \ Hg * 568 \ mL )/(248.71 \ mm \ Hg)`

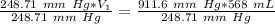
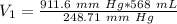
The units of millimeters of mercury (mm Hg) cancel.

`V_1 = (911.6 * 568 \ mL )/(248.71 )`

`V_1 = \frac {517788.8} {248.71 } \ mL`

`V_1 = 2081.897793 \ mL`

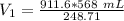
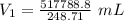
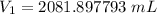
Let's round to the nearest whole number. The 8 in the tenths place tells us to round the 1 up to a 2.

`V_1= 2082 \ mL`

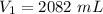
The gas initially occupied a volume of 2082 milliliters and choice A is correct.