Question

In recent years, only 79% of the fruit sold at Fred's Fruit stand has been any good. Fred recently started buying fruit from new farmers and wants to know if there is a statistically significant increase in the percentage of good fruit. In a random sample if 475 pieces, 85 were bad. How would the conclusion change if the significance level went from 7% to 5%?

Answer 1

The p-value of the test is 0.0485 < 0.05, also less than 0.07, so there is sufficient evidence to conclude that there is a statistically significant increase in the percentage of good fruit, for both significance levels, thus the change in significance levels do not change the conclusion.

Explanation:

79% of the fruit sold at Fred's Fruit stand has been any good. Test if there has been an increase.

At the null hypothesis, we test if there has been no increase, that is, the proportion is still of 79%, so:

`H_0: p = 0.79`

At the alternative hypothesis, we test if there has been an increase, that is, more than 79% being good, so:

`H_1: p > 0.79`

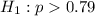
The test statistic is:

`z = (X - \mu)/((\sigma)/(√(n)))`

In which X is the sample mean,
`\mu` is the value tested at the null hypothesis,
`\sigma` is the standard deviation and n is the size of the sample.

0.79 is tested at the null hypothesis:

This means that
`\mu = 0.79, \sigma = √(0.79*0.21)`

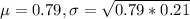
In a random sample if 475 pieces, 85 were bad.

So 475 - 85 = 390 were good, and:

`n = 475, X = (390)/(475) = 0.8211`

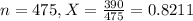
Value of the test statistic:

`z = (X - \mu)/((\sigma)/(√(n)))`

`z = (0.8211 - 0.79)/((√(0.79*0.21))/(√(475)))`

`z = 1.66`

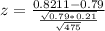
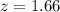
P-value of the test and decision:

The p-value of the test is the probability of finding a sample proportion above 0.8211, which is 1 subtracted by the p-value of Z = 1.66.

Looking at the z-table, z = 1.66 has a p-value of 0.9515.

1 - 0.9515 = 0.0485

The p-value of the test is 0.0485 < 0.05, also less than 0.07, so there is sufficient evidence to conclude that there is a statistically significant increase in the percentage of good fruit, for both significance levels, thus the change in significance levels do not change the conclusion.