Question

A car generator turns at 400 rpm (revolutions per minute) when the engine is idling. It has a rectangular coil with 300 turns of dimensions 5.00 cm by 6.46 cm that rotates in an adjustable magnetic field. What is the field strength needed to produce a 24.0 V peak emf

Answer 1

The field strength needed to produce a 24.0 V peak emf is 0.5913 T

Step-by-step explanation:

From the formula for maximum emf of a generator,

`emf_(max)= NAB\omega`

∴
`B = (emf_(max) )/(NA\omega)`

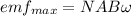
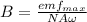
Where N is the number of loops

A is cross section of loops

B is magnetic field strength

ω is the angular frequency of loops

From the question,
`emf_(max) = 24.0V`

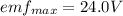
N = 300 turns

A = l × b ( since it is a rectangular coil)

A = 6.46 cm × 5.00 cm

A = 0.0646 m × 0.05 m

A = 0.00323 m²

ω = 400 rpm = (400/60)×2π rad/s

ω = 41.8879 rad/s

Putting all the parameters into the formula

`B = (emf_(max) )/(NA\omega)`

`B = (24.0)/(300*0.00323*41.8879)`

`B = 0.5913T`

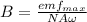
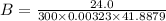
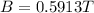
Hence, the field strength needed to produce a 24.0 V peak emf is 0.5913 T