Answer:
A)Let "L" represents lenght and "W" represent the width
L=2W+4
L+W=80
B) 1. W= 80-L
2. L=2(80-L)+4
3. L=160-2L+4
4. L=164-2L
5. 3L=164
6. L=
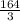 or 54.666
or 54.666
plug in 52 for L back into the original equation to solve W
7.
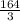 +W= 80
+W= 80
8. W=25.333 or
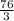
C) The widght is 25.33 feet and the lenght is 55.66 feet