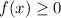Answer:
Domain:

Range:
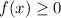
Explanation:
Given
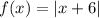
Required
The domain and the range
First, we calculate the domain
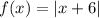
The above function does not have roots or fraction, where x is the denominator. This means that the domain is all real numbers, i.e.

The range
The function is an absolute function; So, the minimum value is 0.
Hence, the range is: