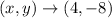Question

Urgent need the answers plz help.

Answer 1

(a)
`P`

(b)
`(x,y) \to (4,-8)`

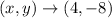
Explanation:

Given

`P = (4,3)`

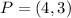
Solving (a): Reflect across x and y-axis.

Reflection across x-axis has the following rules

`(x,y) \to (x,-y)`

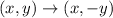
So, we have:

`P' = (4,-3)`

Reflection across y-axis has the following rules

`(x,y) \to (-x,y)`

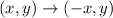
So, we have:

`P`

Hence, the new point is: (-4,-3)

Solving (b): Rx . Do,2 (2,4)

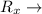`R_x \to` reflect across the x-axis

Reflection across x-axis has the following rules

`(x,y) \to (x,-y)`

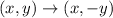
So, we have:

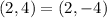`(2,4) = (2,-4)` ---- when P is reflected across the x-axis

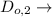`D_(o,2) \to` dilate by a scale factor of 2

The rule is:

`(x,y) \to 2 * (x,y)`

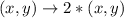
So, we have

`(x,y) \to 2 * (2,-4)`

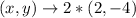
Open bracket

`(x,y) \to (4,-8)`