Explanation:
Step 1: Factor the equation
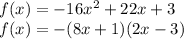
Step 2: Find the x-intercepts of the graph of f(x)
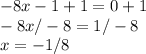
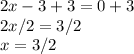
Step 3: Describe the end behavior of the graph of f(x)
Since the function is to the power of 2, that means that it is a parabola. And since the leading coefficient is negative, means that the arrows will be pointing down therefore, the end behavior of this graph is as x goes to infinity, f(x) goes to negative infinity and as x goes to negative infinity, f(x) goes to negative infinity.
Step 4: What are the steps you would use to graph f(x)
The first step that I would do is factor the equation. Then I would find the x-intercepts of the graph and plot them on the graph. I would then plug in 0 for all of the x values to get the y intercept. After doing that I would get the vertex using the vertex formula plotting it on the graph. Finally, I would connect all of the dots together to form the graph of the equation.