Question

In 4 years Craston's age will be the same as Terrins age is now. In 2 years, Terrin will be twice as old as craston. Find their ages now

Answer 1

Answer:2 and 6

Explanation:

Given

In 4 years Craston's age will be the same as Terrins age is now

Suppose the present of Craston's and Terrins are
`x` and
`y` respectively

According to the question

`\Rightarrow x+4=y\\\Rightarrow y-x=4\quad \ldots(i)`

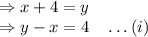
In 2 years Terrin will be twice as old as craston

`\Rightarrow (y+2)=2(x+2)\\\Rightarrow y-2x=4-2\\\Rightarrow y-2x=2\quad \ldots(ii)\\`

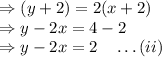
Solving (i) and (ii) we get

`x=2,y=6`

Thus, the present age of Craston and Terrins are
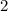`2` and
`6`