Answer:
Given:
- The radius of the driving wheel of an engine is 1.75m
To Find:
- How many revolutions will it make in traveling 22 km.
Solution:
First, we have to find the Circumference of the Wheel:
As, we know:
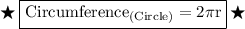
where,
- pi (π) = 22/7
- Radius (r) = 1.75m
So, the Circumference of wheel will be:
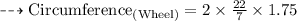
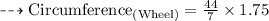
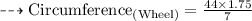
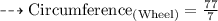
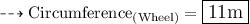
Hence, the circumference of the wheel is 11m.
Now, we have to convert distance covered km into m:
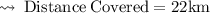
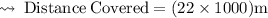
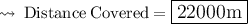
Hence, the distance covered is 22000m.
Now, we have to find the number of revolution:
Given:
- Circumference = 11m
- Distance Covered = 22000m
According to the question by using the formula, we get:
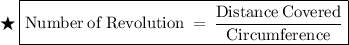
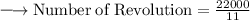
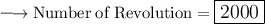
Thus, the revolution will it make in traveling 22km is 2000.