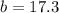Question

Solve the right triangle given that mZA= 30°, mZC = 90°, anda = 15. Round your result to one decimal place.​

Answer 1

`\angle B = 60^o`

`b =17.3`

`c = 20`

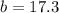
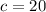
Explanation:

Given

`a= 15`

`\angle A = 30^o`

`\angle C = 90^o`

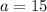
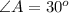
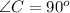
See attachment for illustration

Required

Solve the triangle

First, we calculate the measure of B

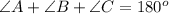`\angle A + \angle B + \angle C = 180^o` --- angles in a triangle

`30^o + \angle B + 90^o = 180^o`

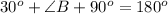
Collect like terms

`\angle B = 180^o-90^o-30^o`

`\angle B = 60^o`

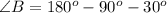
Solve for (c) using sine function

`\sin(30) = (a)/(c)`

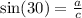
Make c the subject

`c = (a)/(\sin(30))`

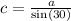
Substitute known values

`c = (10)/(0.5)`

`c = 20`

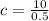
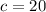
Solve for (b) using Pythagoras

`c^2 = a^2 + b^2`

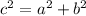
This gives:

`20^2 = 10^2 + b^2`

`400 = 100 + b^2`

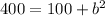
Collect like terms

`b^2 =400 - 100`

`b^2 =300`

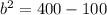
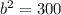
Take square roots

`b =17.3`