Answer:
0.713 = 71.3% probability that the county office will get more than 0 calls in a 15 minute period.
Explanation:
We have the mean during a time-period, which means that the Poisson distribution is used to solve this question.
In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

In which
x is the number of sucesses
e = 2.71828 is the Euler number
 is the mean in the given interval.
is the mean in the given interval.
A county office gets an average of 10 calls in a 2 hour time period.
10 calls each 120 minutes, which means that the mean for n minutes is:
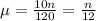
15 minute period:
This means that

What is the probability that the county office will get more than 0 calls in a 15 minute period?
This is:

In which

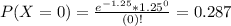
So
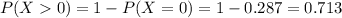
0.713 = 71.3% probability that the county office will get more than 0 calls in a 15 minute period.