Answer:
Step-by-step explanation:
Let assume that the missing aqueous solution of 4-chlorobutanoic acid = 0.76 M
Then, the dissociation of 4-chlorobutanoic acid can be expressed as:
 ⇄
⇄
 +
+

The ICE table can be computed as:
 ⇄
⇄
 +
+

Initial 0.76 - -
Change -x +x +x
Equilibrium 0.76 - x x x
![K_a = \frac{[\mathsf{C_3H_6ClCO_2^-}] [\mathsf{H^+}]}{\mathsf{[C_3H_6ClCO_2H ]}}](https://img.qammunity.org/2022/formulas/chemistry/college/vrt1wop61jl7lbr1b3jfhsbilejik3b6gx.png)
![K_a = ([x] [x])/( [0.76-x])](https://img.qammunity.org/2022/formulas/chemistry/college/zvry30koli33g7agg35f69nlhkzn87hzcj.png)
where:

![3.02*10^(-5) = (x^2)/( [0.76-x])](https://img.qammunity.org/2022/formulas/chemistry/college/3bcf2mu8xkj4x5hm6198xs71zpdi8t8sf5.png)
however, the value of x is so negligible:
0.76 -x = 0.76
Then:
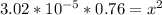

x = 0.00479 M
∴
![x = \mathsf{[C_3H_6ClCO_2^-] = [H^+]=}](https://img.qammunity.org/2022/formulas/chemistry/college/9pd0p2w1lxu9eferinw8ggbbr0jfu35cww.png) 0.00479 M
0.00479 M
 = (0.76 - 0.00479) M
= (0.76 - 0.00479) M
= 0.75521 M
Finally, the percentage of the acid dissociated is;
= ( 0.00479 / 0.76) × 100
= 0.630 M