Question

The length of a rectangle is 8 cm longer than its width. find the dimensions of the rectangle if its area is 108cm

!!no links!!

Answer 1

`4+2√(31)\text{ by } -4+2√(31)`

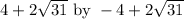
Or about 15.136 centimeters by 7.136 centimeters.

Explanation:

Recall that the area of a rectangle is given by:

`\displaystyle A = w\ell`

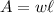
Where w is the width and l is the length.

We are given that the length is 8 centimeters longer than the width. In other words:

`\ell = w+8`

And we are also given that the total area is 108 square centimeters.

Thus, substitute:

`(108)=w(w+8)`

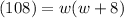
Solve for w. Distribute:

`w^2+8w=108`

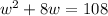
Subtract 108 from both sides:

`w^2+8w-108=0`

Since the equation is not factorable, we can use the quadratic formula:

`\displaystyle x = (-b\pm√(b^2-4ac))/(2a)`

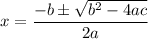
In this case, a = 1, b = 8, and c = -108. Substitute and evaluate:

`\displaystyle \begin{aligned} w&= (-(8)\pm√((8)^2-4(1)(-108)))/(2(1)) \\ \\ &=(-8\pm√(496))/(2)\\ \\ &=(-8\pm4√(31))/(2) \\ \\ &=-4 \pm 2√(31) \end{aligned}`

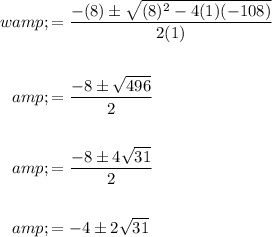
So, our two solutions are:

`w=-4+2√(31) \approx 7.136 \text{ or } w=-4-2√(31)\approx -15.136`

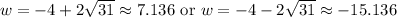
Since width cannot be negative, we can eliminate the second solution.

And since the length is eight centimeters longer than the width, the length is:

`\ell =(-4+2√(31))+8=4+2√(31)\approx 15.136`

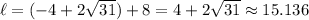
So, the dimensions of the rectangle are about 15.136 cm by 7.136 cm.