Given:
The inequalities are:
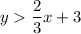
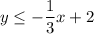
To find:
The graph for the given system of inequities.
Solution:
We have,
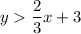
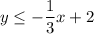
The related equations are:
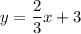

Table of values
x
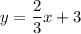

0 3 2
3 5 1
Plot the points (0,3) and (3,5) and connect them by a straight line to get the boundary line
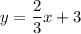 .
.
Plot the points (0,2) and (3,1) and connect them by a straight line to get the boundary line
 .
.
In
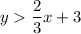 , the sign of inequality is ">" it means the boundary line is a dashed line and shaded area lies above the boundary line.
, the sign of inequality is ">" it means the boundary line is a dashed line and shaded area lies above the boundary line.
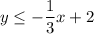 , the sign of inequality is "
, the sign of inequality is "
 " it means the boundary line is a solid line and shaded area lies below the boundary line.
" it means the boundary line is a solid line and shaded area lies below the boundary line.
Therefore, the required graph is shown below.