Answer:
x = (log₅7) - 8
Step-by-step explanation:
Given;
 = 7
= 7
Take log of both sides;
log₁₀(
 ) = log₁₀7 -------------(ii)
) = log₁₀7 -------------(ii)
From the laws of logarithm remember that;
logₐ xⁿ = n logₐ x
Equation (ii) can then be written as;
(x + 8)log₁₀5 = log₁₀7
Divide both sides by log₁₀5
(x + 8) =
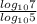 -----------(iii)
-----------(iii)
From the laws of logarithm, remember that;
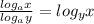
Equation (iii) can thus be written as;
(x + 8) = log₅7
x + 8 = log₅7
Make x subject of the formula;
x = (log₅7) - 8