Question

A 1.25 kg block is attached to a spring with spring constant 17.0 N/m . While the block is sitting at rest, a student hits it with a hammer and almost instantaneously gives it a speed of 49.0 cm/s . What are You may want to review (Pages 400 - 401) . Part A The amplitude of the subsequent oscillations

Answer 1

The amplitude of the subsequent oscillations is 13.3 cm

Step-by-step explanation:

Given;

mass of the block, m = 1.25 kg

spring constant, k = 17 N/m

speed of the block, v = 49 cm/s = 0.49 m/s

To determine the amplitude of the oscillation.

Apply the principle of conservation of energy;

maximum kinetic energy of the stone when hit = maximum potential energy of spring when displaced

`K.E_(max) = U_(max)\\\\(1)/(2) mv^2 = (1)/(2) kA^2\\\\where;\\\\A \ is \ the \ maximum \ displacement = amplitude \\\\mv^2 = kA^2\\\\A^2 = (mv^2)/(k) \\\\A = \sqrt{(mv^2)/(k)} \\\\A = \sqrt{(1.25\ * \ 0.49^2)/(17)} \\\\A = 0.133 \ m\\\\A = 13.3 \ cm`

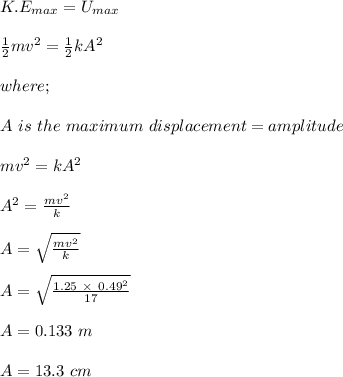
Therefore, the amplitude of the subsequent oscillations is 13.3 cm