Complete question is: While running, a person dissipates about 0.60 J of mechanical energy per step per kilogram of body mass. If a 60-kg person develops a power of 70 W during a race, how fast is the person running? (Assume a running step is 1.5 m long).
Answer: The person running at a speed of 2.91 m/s.
Step-by-step explanation:
Given: Mass of runner = 60 kg
Runner dissipates = 0.6 J/kg per step
Average power = 70 W
1 step = 1.50 m
Energy dissipated by the runner is as follows.

Formula used to calculate the value of one step 'S' is as follows.
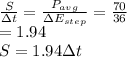
It is known that average velocity is equal to the total distance divided by time interval.
So, total distance for the given situation is as follows.
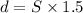
Hence, speed of the person is calculated as follows.

Thus, we can conclude that the person running at a speed of 2.91 m/s.