Answer:
The kinetic energy of the electron just before the collision is 9.3 eV.
Step-by-step explanation:
We can find the kinetic energy of the electron before the collision can be found by energy conservation:

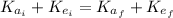 (1)
(1)
Where:
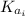 : is the initial kinetic energy of the atom
: is the initial kinetic energy of the atom
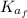 : is the final kinetic energy of the atom = 6.1 eV +
: is the final kinetic energy of the atom = 6.1 eV +
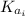
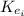 : is the initial kinetic energy of the electron =?
: is the initial kinetic energy of the electron =?
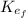 : is the final kinetic energy of the electron = 3.2 eV
: is the final kinetic energy of the electron = 3.2 eV
By solving equation (1) for
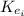 we have:
we have:

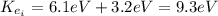
Therefore, the kinetic energy of the electron just before the collision is 9.3 eV.
I hope it helps you!