Answer:
Explanation:
Begin by dividing both sides by -4 to get:
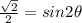 and then take the inverse sin of both sides to get
and then take the inverse sin of both sides to get
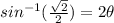 (the inverse sin undoes the sin on the right). Now we need to look on our unit circle to find the angles where
(the inverse sin undoes the sin on the right). Now we need to look on our unit circle to find the angles where
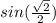 is positive. There are 2 places. Sin is positive in both QI and QII. The angles are
is positive. There are 2 places. Sin is positive in both QI and QII. The angles are
 . Therefore, our 2 equations are
. Therefore, our 2 equations are
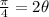 and
and
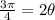 . Solving the first equation:
. Solving the first equation:
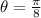
and the second equation:
