Answer:
When the number of rows or number of columns in the given matrix does not match the number of entries in the vector x.
When the number of columns in A
 the number of rows in B.
the number of rows in B.
The matrix-vector is not defined.
Explanation:
For example:-
Step 1:
Let's assume
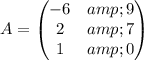 3x2 &
3x2 &
 3x1
3x1
Since matrix multiplication of matric A and matrix B is only possible,
When the number of columns in A = Number of rows in B.
Step 2:
Let's A as m x n; Here m = rows and n=columns
B as p x q; Here p = rows and q=columns.
When n
 p, the matrix-vector is not defined.
p, the matrix-vector is not defined.
That is matrix multiplication Ax is not possible.
Hence the matrix is not defined.