Question

asked Oct 21, 2022 160k views

2 Answers

Maressyl · Answer 1 · 2022-10-22T16:43:41+0000

___________________________________

Problem:

$\quad\quad\quad\quad\tt{A.) x\tiny{1}\small{=-8}, y\tiny{1}\small{=4}}$

$\quad\quad\quad\quad\tt{B.) x\tiny{2}\small{=4}, y\tiny{2}\small{=-1}}$

$\quad\quad\quad\quad\tt{d = \sqrt{(x \tiny{2} \small{ - x \tiny{1} \small {)}^(2) + (y \tiny{2} \small{ - y \tiny{1} \small{)}^(2) } }}}$

$\quad\quad\quad\quad\tt{d = \sqrt{(4 - \small{ (- 8}{))}^(2) + ( \small{- 1)}\small{ - {4)}}^(2) }}$

$\quad\quad\quad\quad\tt{d = \sqrt{ ( {12)}^(2) + {( -5)}^(2) }}$

$\quad\quad\quad\quad\tt{d = \sqrt{ {144} + {25}}}$

$\quad\quad\quad\quad\tt{d = √( 169)}$

$\quad\quad\quad\quad\tt{d = 13}$

$\quad\quad\quad\quad\boxed{\boxed{\tt{\color{magenta}d = 13}}}$

___________________________________

#CarryOnLearning

✍︎ C.Rose❀

PavelGP · Answer 2 · 2022-10-27T16:42:15+0000

Answer:

The distance between A(-8, 4) and B(4, -1) is 13 units.

Explanation:

To find the distance between any two points, we can use the distance formula given by:

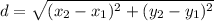
$\displaystyle d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2$

We have the two points A(-8, 4) and B(4, -1). Let A(-8, 4) be (x₁, y₁) and let B(4, -1) be (x₂, y₂). Substitute:

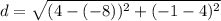
d=√((4-(-8))^2+(-1-4)^2)

Evaluate:

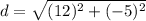
d=√((12)^2+(-5)^2)

So:

$d=√(144+25)=√(169)=13\text{ units}$

The distance between A(-8, 4) and B(4, -1) is 13 units.