Question

A combination lock has a 1.1-cmcm-diameter knob that is part of the dial you turn to unlock the lock. To turn that knob, you grip it between your thumb and forefinger with a force of 0.67 NN as you twist your wrist. Suppose the coefficient of static friction between the knob and your fingers is only 0.14 because some oil accidentally got onto the knob.

Required:
What is the most torque you can exert on the knob without having it slip between your fingers?

Answer 1

1.0318 × 10⁻³ N m

Step-by-step explanation:

From the given question;

The torque can be determined by using the formula:

Torque = Force × radius

where;

Force = Normal force(N) × coefficient of friction (μ)

Force = 0.67 N × 0.14

Force = 0.0938 N

Since force is applied to the knob in two directions;

The Torque of the thumb and the finger can be computed as:

`T_(thumb) =0.0938 *0.55 = 0.05159\ N \ cm \\ \\ T_(finger) =0.0938 *0.55 = 0.05159\ N \ cm`

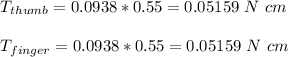
The total max torque = 0.05159 N cm + 0.05159 N cm

= 0.10318 N cm

= 1.0318 × 10⁻³ N m