Question

Which could be a graph which represents a quadratic that is a perfect square trinomial?

Answer 1

The top choice.

Explanation:

If a quadratic function is a perfect square trinomial, its graph will intersect the x-axis exactly once.

`y=(x+1)^2\\`

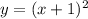
Expanded, this is
`y=(x+1)(x+1)`

There is only one value of x which makes the "two" factors equal to zero, and that is -1, where the graph touches the x-axis.

See the attached graph.
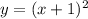`y=(x+1)^2` is the purple graph.

The green graph is an example of a quadratics which is NOT a perfect square. It's the function
`y=x^2-x-2`.