Question

A manufacturer considers his production process to be out of control when defects exceed 3%. In a random sample of 100 items, the defect rate is 4% but the manager claims that this is only a sample fluctuation and production is not really out of control. At the 0.01 level of significance, test the manager's claim.

Answer 1

The p-value of the test is of 0.2776 > 0.01, which means that the we accept the null hypothesis, that is, the manager's claim that this is only a sample fluctuation and production is not really out of control.

Explanation:

A manufacturer considers his production process to be out of control when defects exceed 3%.

At the null hypothesis, we test if the production process is in control, that is, the defective proportion is of 3% or less. So

`H_0: p \leq 0.03`

At the alternate hypothesis, we test if the production process is out of control, that is, the defective proportion exceeds 3%. So

`H_1: p > 0.03`

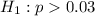
The test statistic is:

`z = (X - \mu)/((\sigma)/(√(n)))`

In which X is the sample mean,
`\mu` is the value tested at the null hypothesis,
`\sigma` is the standard deviation and n is the size of the sample.

0.03 is tested at the null hypothesis

This means that
`\mu = 0.03, \sigma = √(0.03*0.97)`

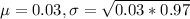
In a random sample of 100 items, the defect rate is 4%.

This means that
`n = 100, X = 0.04`

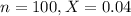
Value of the test statistic:

`z = (X - \mu)/((\sigma)/(√(n)))`

`z = (0.04 - 0.03)/((√(0.03*0.97))/(√(100)))`

`z = 0.59`

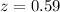
P-value of the test

The p-value of the test is the probability of finding a sample proportion above 0.04, which is 1 subtracted by the p-value of z = 0.59.

Looking at the z-table, z = 0.59 has a p-value of 0.7224

1 - 0.7224 = 0.2776

The p-value of the test is of 0.2776 > 0.01, which means that the we accept the null hypothesis, that is, the manager's claim that this is only a sample fluctuation and production is not really out of control.