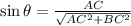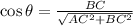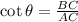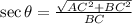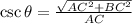Answer:
The trigonometric ratios are presented below:
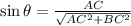
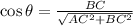
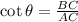
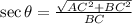
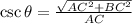
Explanation:
From Trigonometry we know the following definitions for each trigonometric ratio:
Sine
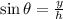 (1)
(1)
Cosine
 (2)
(2)
Tangent
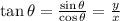 (3)
(3)
Cotangent
 (4)
(4)
Secant
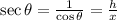 (5)
(5)
Cosecant
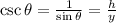 (6)
(6)
Where:
 - Adjacent leg.
- Adjacent leg.
 - Opposite leg.
- Opposite leg.
 - Hypotenuse.
- Hypotenuse.
The length of the hypotenuse is determined by the Pythagorean Theorem:

If
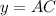 and
and
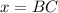 , then the trigonometric ratios are presented below:
, then the trigonometric ratios are presented below: