Answer:
19 terms
Explanation:
the sum to n terms of an AP is
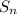 =
=
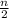 [ 2a₁ + (n - 1)d ]
[ 2a₁ + (n - 1)d ]
where a₁ is the first term and d the common difference
here a₁ = 18 and d = a₂ - a₁ = 16 - 18 = - 2, then solving for n
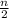 [ (2 × 18) - 2(n - 1) ] = 0 ( multiply both sides by 2 to clear the fraction )
[ (2 × 18) - 2(n - 1) ] = 0 ( multiply both sides by 2 to clear the fraction )
n(36 - 2n + 2) = 0
n(38 - 2n) = 0 ← distribute parenthesis on left side by n
38n - 2n² = 0 ← factor out 2n from each term on the left
2n(19 - n) = 0
equate each factor to zero and solve for n
2n = 0 ⇒ n = 0
19 - n = 0 ⇒ n = 19
n > 0 , then number of terms is 19