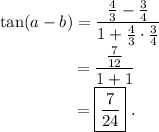Answer:
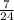
Explanation:
By the Pythagorean Theorem, we know
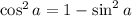 .
.
With this, we can find
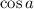 by plugging in what we know for
by plugging in what we know for
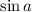 :
:
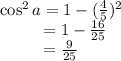 .
.
Taking the square root, we get
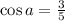 .
.
Note: there seems to be a problem with the question? It doesn't specify what range
 lies in, so we don't know whether
lies in, so we don't know whether
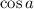 is positive or negative. In this case, I assumed it was positive.
is positive or negative. In this case, I assumed it was positive.
From this, we can find
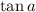 :
:
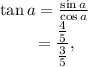
so
 .
.
Using the
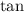 difference formula, we know
difference formula, we know
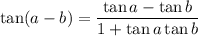 .
.
Plugging in the values we know for
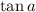 and
and
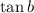 , we get
, we get