Answer:
The speed of the bullet just before the impact is 701 m/s
Step-by-step explanation:
Given;
mass of the bullet, m₁ = 20 g = 0.02 kg
mass of the block, m₂ = 2.8 kg
displacement of the block, d = 9 mm = 9 x 10⁻³ m
duration of motion of the bullet, t = 5 ms = 5 x 10⁻³ s
Apply the principle of conservation of energy;
The final kinetic energy of the bullet = maximum potential energy of the block
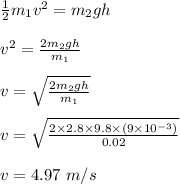
Apply the principle of conservation of linear momentum, to determine the initial velocity of the bullet before the impact.
m₁u₁ + m₂u₂ = v(m₁ + m₂)
where;
u₁ is the initial velocity of the bullet
u₂ is the initial velocity of the block = 0
m₁u₁ + 0 = v(m₁ + m₂)
m₁u₁ = v(m₁ + m₂)
0.02u₁ = 4.97(2.8 + 0.02)
0.02u₁ = 14.02
u₁ = 14.02 / 0.02
u₁ = 701 m/s
Therefore, the speed of the bullet just before the impact is 701 m/s