Question

Find the surface area, and please show me steps so i can understand this myself !

Answer 1

The surface area of the triangular prism is approximately 401.582 square feet.

Explanation:

The figure represents a triangular prism, the procedure for calculating the surface area of the entire figure consists in adding the areas of each face (3 rectangles and 2 triangles):

`A = 2\cdot \left[(1)/(2)\cdot (9\,ft)\cdot (4\,ft)\right] + (16\,ft)\cdot (4\,ft) + (9\,ft)\cdot (16\,ft) + (16\,ft)\cdot \sqrt{(9\,ft)^(2)+(4\,ft)^(2)}`
`A \approx 401.582\,ft^(2)`

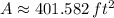
The surface area of the triangular prism is approximately 401.582 square feet.