Question

A person slaps her leg with her hand, which results in her hand coming to rest in a time interval of 2.65 ms from an initial speed of 5.25 m/s . What is the magnitude of the average contact force exerted on the leg, assuming the total mass of the hand and the forearm to be 1.75 kg

Answer 1

the magnitude of the average contact force exerted on the leg is 3466.98 N

Step-by-step explanation:

Given the data in the question;

Initial velocity of hand v₀ = 5.25 m/s

final velocity of hand v = 0 m/s

time interval t = 2.65 ms = 0.00265 s

mass of hand m = 1.75 kg

We calculate force on the hand F
`_{hand`

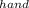
using equation for impulse in momentum

F
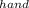`_{hand` × t = m( v - v₀ )

we substitute

F
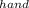`_{hand` × 0.00265 = 1.75( 0 - 5.25 )

F
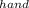`_{hand` × 0.00265 = 1.75( - 5.25 )

F
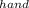`_{hand` × 0.00265 = -9.1875

F
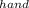`_{hand` = -9.1875 / 0.00265

F
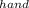`_{hand` = -3466.98 N

Next we determine force on the leg F
`_{leg`

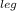
Using Newton's third law of motion

for every action, there is an equal opposite reaction;

so, F
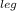`_{leg` = - F
`_{hand`

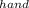
we substitute

F
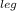`_{leg` = - ( -3466.98 N )

F
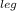`_{leg` = 3466.98 N

Therefore, the magnitude of the average contact force exerted on the leg is 3466.98 N