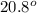Question

Practice 4b

In the diagram, PQRS is a rectangular sloping surface, PQTU is a rectangle on horizontal ground and RI and
SU are vertical lines. PQ = SR=UT = 45 cm, QR = PS = 32 cm and angle RQT= 38º.

Calculate
PR,
(b) RT, and
(c) angle RPT

Answer 1

(a) PR = 55.2 cm

(b) RT = 19.7 cm

(c) <RPT =
`20.8^(o)`

Explanation:

(a) to determine the value of PR, apply the Pythagoras theorem to PQR.

PQ = 45, and SP = QR = 32. So that;

`/hyp/^(2)` =
`/Adj 1/^(2)` +
`/Adj 2/^(2)`

`/PR/^(2)` =
`45^(2)` +
`32^(2)`

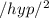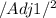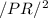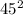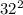
= 2025 + 1024

= 3049

PR =
`√(3049)`

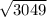
= 55.218

PR = 55.2 cm

(b) To determine RT, apply the appropriate trigonometric function to QRT.

Let RT be represented by x, so that;

Sin
`38^(o)` =
`(x)/(32)`

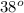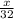
x = 32 * Sin
`38^(o)`

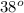
= 32 * 0.6157

x = 19.7024

RT = 19.7 cm

(c) To determine <RPT, let the angle be represented by θ.

Sin θ =
`(opposite)/(hypotenuse)`

=
`(RT)/(PR)`

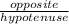
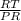
Sin θ =
`(19.7024)/(55.218)`

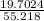
= 0.3568

θ =
`Sin^(-1)` 0.35568

=
`20.84^(o)`

Thus, <RPT =
`20.8^(o)`