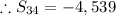Answer:
-4,539
Explanation:
Given series is: 147 + 130 + 113 + 96 + . . .
Where first term a = 147
Common Difference d = 130 - 147 = - 17
No. of terms n = 34
To find:

By sum of n terms of an Arithmetic Progression, we have:
![S_(n)=(n)/(2)[2a + (n-1)d]](https://img.qammunity.org/2022/formulas/mathematics/college/y6l81ucgpp2tqmsznn8wol7u0wio18yle5.png)
Plugging the values of a, d and n in the above equation, we find:
![S_(34)=(34)/(2)[2(147) + (34-1)(-17)]](https://img.qammunity.org/2022/formulas/mathematics/college/70fyvrxlw7amhkreokrv07mzj5x06z3xr3.png)
![\therefore S_(34)=17[294 + (33)(-17)]](https://img.qammunity.org/2022/formulas/mathematics/college/2eumh7gs1jlp0f19hgdisyu0gkxfumwrtg.png)
![\therefore S_(34)=17[294 - 561]](https://img.qammunity.org/2022/formulas/mathematics/college/58sdiisq32xu4yl2hdvh8p6k5p2t6uur9z.png)
![\therefore S_(34)=17[-267]](https://img.qammunity.org/2022/formulas/mathematics/college/84990bz16anb6o6k3cq2ammakqxdj9biaf.png)