Question

Using the picture above Find the measure of arc EF

Answer 1

Given:

Measure of arc CG is 125 degrees.

The measure of angle CDG is 85 degrees.

To find:

The measure of arc EF.

Solution:

Intersecting chords theorem: If two chords intersect each other inside the circle, then the measure of angle on the intersection is half of the sum of intercepted arcs.

Using the intersecting chords theorem, we get

`m\angle CDG=(1)/(2)(m(arcCG)+m(arcEF))`

Let x degrees be the measure of arc EF.

Substituting the given values, we get

`85^\circ=(1)/(2)(125^\circ+x)`

`2* 85^\circ=125^\circ+x`

`170^\circ -125^\circ=x`

`45^\circ=x`

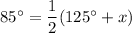
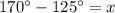
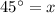
Therefore, the measure of arc EF is
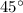`45^\circ`.