Answer:
1. The magnitude of the net force is 1.33 N.
2. The direction is 14.8° with respect to the vertical.
Step-by-step explanation:
1. The magnitude of the net force is given by:

Where:
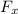 : is the sum of the forces acting in the x-direction
: is the sum of the forces acting in the x-direction
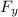 : is the sum of the forces acting in the y-direction
: is the sum of the forces acting in the y-direction
Let's find the forces acting in the x-direction and in the y-direction.
In the x-direction:
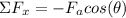
Where:
Fa: is the force of air resistance = -0.40 N. The negative sign is because this force is in the negative x-direction.
θ: is the angle = 32°

In the y-direction:
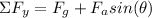
Where:
 : is the gravitational force = -1.5 N
: is the gravitational force = -1.5 N

Hence, the magnitude of the net force is:
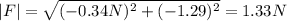
2. The direction of the net force is:
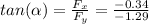
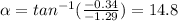
The angle is 14.8° with respect to the vertical.
I hope it helps you!