Question

What is the quotient of 1.888\times 10^91.888×10 9 and 5.9 \times 10^65.9×10 6 expressed in scientific notation?

Answer 1

Given:

The expressions are
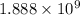`1.888* 10^9` and
`5.9* 10^6`.

To find:

The quotient of
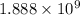`1.888* 10^9` and
`5.9* 10^6` expressed in scientific notation.

Solution:

Quotient of
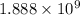`1.888* 10^9` and
`5.9* 10^6` is:

`(1.888* 10^9)/(5.9* 10^6)=(1.888)/(5.9)* (10^9)/(10^6)`

`(1.888* 10^9)/(5.9* 10^6)=0.32* 10^(9-6)`

`(1.888* 10^9)/(5.9* 10^6)=0.32* 10^(3)`

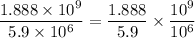
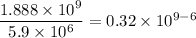
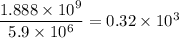
In the scientific notation, the first number is between 1 to 10. So, 0.32 can be written as
`(3.2)/(10)`.

`(1.888* 10^9)/(5.9* 10^6)=(3.2)/(10)* 10^(3)`

`(1.888* 10^9)/(5.9* 10^6)=3.2* 10^(3-1)`

`(1.888* 10^9)/(5.9* 10^6)=3.2* 10^(2)`

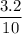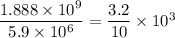
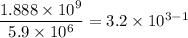
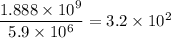
Therefore, the value of the quotient is
`3.2* 10^(2)`.