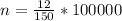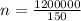Question

If the homes are representative of the community of 100,000 homes that the company serves about how many homes would you predict have 4 or more telephones?

Answer 1

8000 homes

Explanation:

Given

See attached for result of the survey

Required

Number of homes to have 4 or more telephones in 100000

First, we calculate the proportion of homes with 4 or more telephones

`Pr = (4\ or\ More)/(Total)`

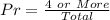
So, we have:

`Pr = (12)/(7+33+56+42+12)`

`Pr = (12)/(150)`

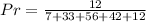
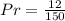
Multiply this by 100000 to get the predicted value

`n = Pr *100000`

`n = (12)/(150) * 100000`

`n = (1200000)/(150)`

`n = 8000`