Answer:
not balanced, oxygen, not equal
Step-by-step explanation:
Alright, so let's go ahead and balance this equation!
The easiest way to do this is to take out a piece of paper and write down your elements on each side. Let's do it together:
--------------------------------------------------------------------------------------------------------------
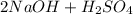 →
→
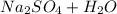
Let's count the number of molecules for each element on both sides. Starting with the left side:
↓
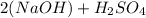
The coefficient of 2 in front of NaOH will be distributed to Na, O, and H. The subscripts for H and O only apply to themselves, so S will have only one molecule. Then our molecule counts for the reactant side (the left side) will be:
↓
Na: 2
O: 2+4 = 6
H: 2+2 = 4
S: 1
↓
Now, let's move on to the right side:
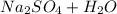
This side only has subscripts, no coefficients, so that means that any elements that don't have subscripts (S and the second O ) will have a count of one. Knowing this, our molecule count for the product side (right side) is:
↓
Na: 2
O: 4+1 = 5
H: 2
S: 1
↓
Let's compare our counts now:
Left side: Right side:
Na: 2 Na: 2
O: 6 O: 5
H: 4 H: 2
S: 1 S: 1
We can see that our O and H molecule counts are different. So that means it's an unbalanced equation.
--------------------------------------------------------------------------------------------------------------
Therefore, your answer will be:
The equation is not balanced because the number of hydrogen atoms and oxygen atoms is not equal in the reactants and in the products.