Question

A study conducted by a group of yoga professionals claim that introducing meditation activities in workplace can significantly increase the performance of the employees. After finding out about this​ study, the HR department of company ABC introduces similar activities in their office and encourages every employee to participate. At the end of one month the department is interested in checking the claims of the study. The department selects a random sample of employees and collects information on the number of hours they have spent meditating ​(X​) and they score their performances based on their revenue contribution to the company ​(​Yi). They estimate the following regression​ function: ​

^Y = 45.63 + 3.60X
where ^Y denotes the predicted value of the score obtained by the individual and X denotes the number of hours they spend meditating. The SE​(​^P1) is 1.01. The HR department wishes to test whether or not meditating increases the performance of their employees. Which of the following are the null and the alternative hypotheses of the test the department wishes to​ conduct?
A. H0: beta1 = 0 vs H1: beta1 is not equal to 0.
B. H0: beta1 = 0 vs H1: beta1 < 0.
C. H0: beta1 = 3.60 vs H1: beta is not equal to 3.60.
D. H0: beta1 = 0 vs H1: beta1 > 0.
A. The value of the t​-statistic associated with the test the HR department wishes to conduct is____.
At the​ 5% significance​ level, the department would____the null hypothesis.

Answer 1

D

T= 3.56

Reject

Explanation:

The estimated equation of an employee's commitment to the business (Y) based on the amount of hours they spend meditating (X) is:

`\hat Y = 45.63 + 3.60 *X`

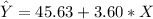
Also;

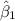`\hat \beta_1` the coefficient of X from the equation Y on X = 3.60

Standard error S.E
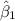`\hat \beta_1` = 1.01

The null & alternative hypothesis:

`H_o : \beta_1 = 0 \\ \\ H_1 : \beta > 0`

Test statistics:

`T = (\hat \beta _1)/(S.E (\hat \beta _1))`

`T = (3.60)/(1.01)`

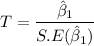
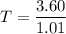
T = 3.56

Value of T-statistics = 3.56

P-value = P(t > T)

here;

t = standard t variable with a degree of freedom = 100 - 1 = 99

∴

P value = P(t > 3.56)

P value = 1 - P(t < 3.56)

P value = 1 - 0.9997

P value = 0.0003

Since p-value is less than ∝ at 0.05

We reject the
`H_o` (null hypothesis)