Question

The proportion of adults who own a cell phone in a certain Canadian city is believed to be 65%. Forty adults are to be selected at random from the city. Let Xbe the number in the sample who own a cell phone. Under the assumptions given, the distribution of X is

A. Bin(40,0.65)
B. N(26,14)
C. Bin(40,26)
D. N(40,14)

Answer 1

The distribution of the number of adults in a sample who own a cell phone, given a 65% ownership rate in the population and a sample size of 40, is Bin(40, 0.65).

Step-by-step explanation:

The proportion of adults in a certain Canadian city who own a cell phone is assumed to be 65%. If we randomly select 40 adults from this city, the random variable X - the number of adults in the sample who own a cell phone - will follow a binomial distribution. This is because the selection of each adult is an independent event with only two outcomes (owning or not owning a cell phone), and the probability of each adult owning a cell phone is the same (65%).

Therefore, the distribution of X is described as a binomial distribution with the number of trials, n, equal to 40 (the number of adults selected), and the probability of success, p, equal to 0.65 (the proportion of cell phone owners). This distribution is denoted as Bin(40, 0.65).

Answer

The distribution of X is A. Bin(40,0.65).

Answer 2

A. Bin(40,0.65)

Step-by-step explanation:

For each adult, there are only two possible outcomes. Either they have a cell phone, or they do not. The probability of an adult having a cell phone is independent of any other adult having a cell phone. This means that the binomial probability distribution is used to solve this question.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

In which
`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

And p is the probability of X happening.

In function of its parameters, the distribution is written as: Bin(n,p).

The proportion of adults who own a cell phone in a certain Canadian city is believed to be 65%

This means that
`p = 0.65`

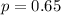
Forty adults are to be selected at random from the city.

This means that
`n = 40`.

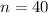Thus, we have Bin(40,0.65), and the correct answer is given by option A.