Question

A recent survey by nerdwallet indicated Americans paid a mean of $6,658 interest on credit card debt in 2017. A sample of 12 households with children revealed the following amounts. 7,077 5,744 6,753 7,381 7,625 6,636 7,164 7,348 8,060 5,848 9,275 7,052 Click here for the Excel Data File State the null hypothesis and the alternate hypothesis. State the decision rule for 0.05 significance level. (Round your answer to 3 decimal places.) Compute the value of the test statistic. (Round your answer to 3 decimal places.) Is it reasonable to conclude that these households paid more interest than the survey result

Answer 1

The null hypothesis is
`H_0: \mu = 6658`

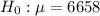
Tha alternate hypothesis is
`H_a: \mu > 6658`

The decision rule is that if
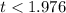`t < 1.976`, the null hypothesis is not rejected, that is, there is no evidence to conclude that these households paid more interest than the survey result, and that if
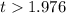`t > 1.976`, the null hypothesis is reject and there is evidence to conclude that these households paid more interest than the survey result.

The test statistic is
`t = 1.94`

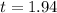
As the test statistic of
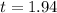`t = 1.94` is higher than the critical value of t, it is reasonable to conclude that these households paid more interest than the survey result

Explanation:

A recent survey by nerdwallet indicated Americans paid a mean of $6,658 interest on credit card debt in 2017. Test if it is reasonable to conclude that these households paid more interest than the survey result.

At the null hypothesis, we test if they paid the same, that is:

`H_0: \mu = 6658`

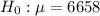
At the alternate hypothesis, we test if they paid more, that is:

`H_a: \mu > 6658`

Test statistic:

The test statistic is:

`t = (X - \mu)/((s)/(√(n)))`

In which X is the sample mean,
`\mu` is the value tested at the null hypothesis, s is the standard deviation of the sample and n is the size of the sample.

State the decision rule for 0.05 significance level.

Right-tailed test(test if the mean is higher than the value) with 12 - 1 = 11 degrees of freedom, has a critical value of t = 1.976.

So

The decision rule is that if
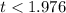`t < 1.976`, the null hypothesis is not rejected, that is, there is no reasonable evidence to conclude that these households paid more interest than the survey result, and that if
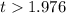`t > 1.976`, the null hypothesis is reject and there is reasonable evidence to conclude that these households paid more interest than the survey result.

6658 is tested at the null hypothesis:

This means that
`\mu = 6658`

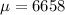
Sample mean and standard deviation:

With the given data-set:

Mean of
`X = 7163.6`

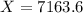
Standard deviation of
`s = 902.25`

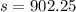
Sample of 12:

This means that
`n = 12`

Compute the value of the test statistic.

`t = (X - \mu)/((s)/(√(n)))`

`t = (7163.6 - 6658)/((902.25)/(√(12)))`

`t = 1.94`

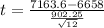
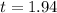
As the test statistic of
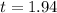`t = 1.94` is higher than the critical value of t, it is reasonable to conclude that these households paid more interest than the survey result