Answer:
x = 12
Explanation:
Since the 2 legs and base angles are congruent the triangle is isosceles.
The line from the vertex to the base is a perpendicular bisector dividing the triangle into 2 right triangles.
Using Pythagoras' identity in either of the 2 right triangles with legs 3 and
 x , hypotenuse
x , hypotenuse
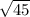 , then
, then
(
 x )² + 3² = (
x )² + 3² = (
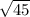 )²
)²
 x² + 9 = 45 ( subtract 9 from both sides ²)
x² + 9 = 45 ( subtract 9 from both sides ²)
 x² = 36 ( multiply both sides by 4 to clear the fraction )
x² = 36 ( multiply both sides by 4 to clear the fraction )
x² = 144 ( take the square root of both sides )
x =
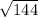 = 12
= 12