Answer:
1) Use translation to coincide the center of a circle with the center of the other circle.
2) We construct the respective loci for the circles.
3) Compare each loci by direct inspection (
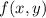 must be the same and radii must be different but constant) to conclude the similarity of the two circles.
must be the same and radii must be different but constant) to conclude the similarity of the two circles.
Explanation:
Geometrically speaking, a circle is formed after knowing its center and radius.
1) Use translation to coincide the center of a circle with the center of the other circle. In this case, we translate the center (
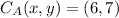 ) of the circle A to the location of the center of the circle B (
) of the circle A to the location of the center of the circle B (
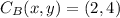 ):
):
![C_(A') (x,y) = (6,7) + [(2,4) - (6,7)]](https://img.qammunity.org/2022/formulas/mathematics/high-school/5bxdegqx2fqmkm1lbheuydvg5qhwb8ep4k.png)
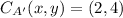
2) We construct the respective loci for the circles.
By Analytical Geometric, a circle is represented by the following locus:
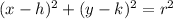
Where:
 - Coordinates of a point of the circunference.
- Coordinates of a point of the circunference.
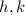 - Coordinates of the center of the circle.
- Coordinates of the center of the circle.
 - Radius of the circle.
- Radius of the circle.
3) Compare each loci by direct inspection (
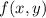 must be the same and radii must be different but constant) to conclude the similarity of the two circles.
must be the same and radii must be different but constant) to conclude the similarity of the two circles.
Then, the circles A' and B are represented by the following loci:
Circle A'
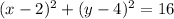
Circle B
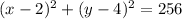
Since both the
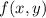 component of each circle is the same and radii are different but constant, then we conclude that circle A is similar to circle B.
component of each circle is the same and radii are different but constant, then we conclude that circle A is similar to circle B.