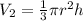Question

Since the area of the circle is I the area of the square, the

volume of the cone equals

o

I the volume of the pyramid or

(ench

or

srh.

o

the volume of the pyramid or (2n
o

the volume of the pyramid or

(2nm or s?h.

o

the volume of the pyramid or : ( 20%(h) or şir?h.

Answer 1

(b)

`V_2 = (\pi)/(4) [ ((2r)^2h)/(3)]` or
`V_2 = (1)/(3)\pi r^2h`

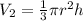
Explanation:

Given

`Ratio = (\pi)/(4)`

See attachment for complete question

Required

Determine the volume of the cone

The volume of a square pyramid is:

`V = (a^2h)/(3)`

Where

a = base dimension

From the attachment, the base dimension of the square pyramid is 2r.

So:

`a = 2r`

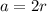
The volume becomes;

`V = ((2r)^2h)/(3)`

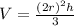
To calculate the volume of the cone, we simply multiply the given ratio and the volume of the prism.

So, we have:

`V_2 = Ratio * V`

`V_2 = (\pi)/(4) [ ((2r)^2h)/(3)]`

`V_2 = (\pi)/(4) * ((2r)^2h)/(3)`

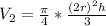
Open bracket;

`V_2 = (\pi)/(4) * (4r^2h)/(3)`

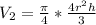
Cancel out 4

`V_2 = \pi * (r^2h)/(3)`

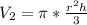
The above can be written as:

`V_2 = (1)/(3) * \pi r^2h`

`V_2 = (1)/(3)\pi r^2h`

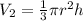
So, we have:

`V_2 = (\pi)/(4) [ ((2r)^2h)/(3)]`

or

`V_2 = (1)/(3)\pi r^2h`